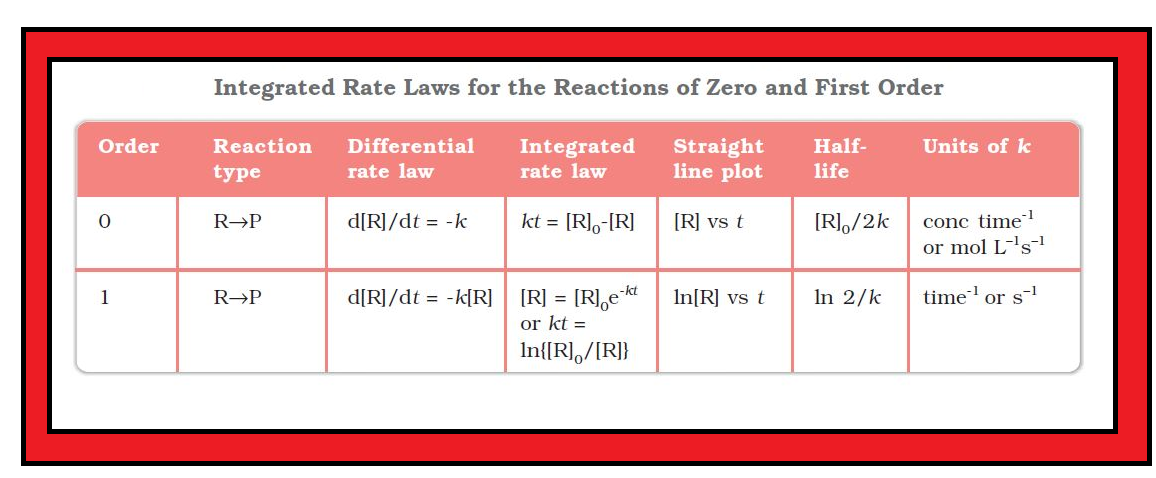
Zero Order Reactions :
`color{green}("Definition") :` Zero order reaction means that the rate of the reaction is proportional to zero power of the concentration of reactants.
Consider the reaction :
`color{red}(R → P)`
`color{red}(text(Rate) = (- d [ R])/(dt) = k [R]^0)`
As any quantity raised to power zero is unity
`color{red}(text(Rate) = - ( d [R])/(dt) = k xx 1)`
`color{red}(d [R] = - k dt)`
Integrating both sides
`color{red}([R] = - k t +I)` ...............(1)
where, `color{red}(I)` is the constant of integration.
At `color{red}(t = 0),` the concentration of the reactant `color{red}(R = [R]_0)`, where `color{red}([R]_0)` is initial concentration of the reactant. Substituting in equation (1)
`color{red}([R]_0 = - k xx 0 + I)`
`color{red}([R]_0 = I)`
Substituting the value of `color{red}(I)` in in the equation (1)
`color{red}([R] = - k t + [R]_0)` ............(2)
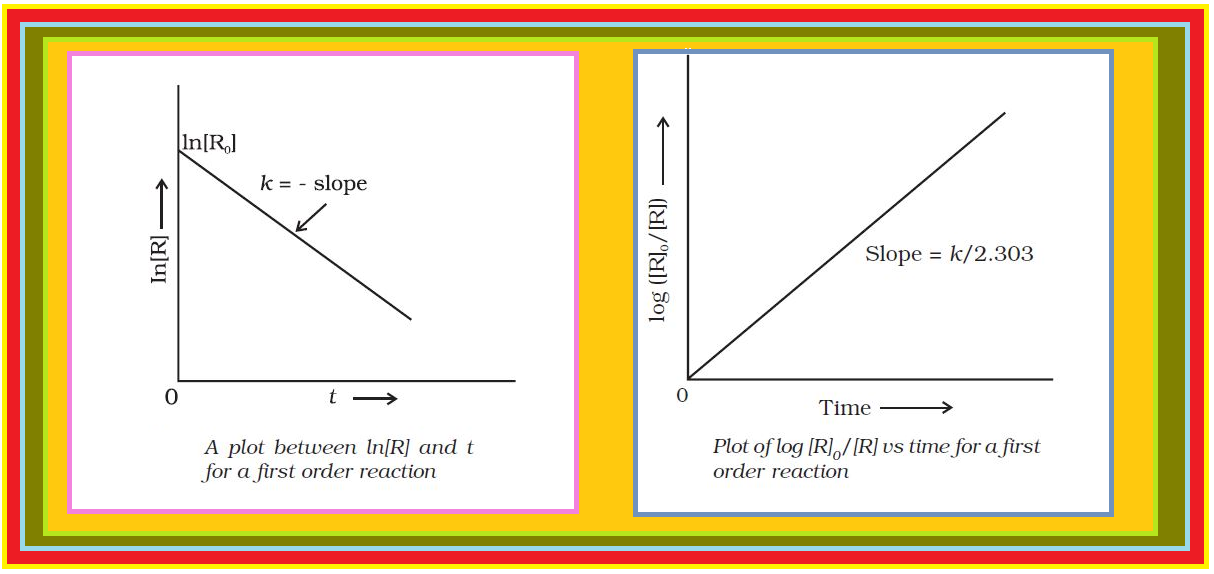
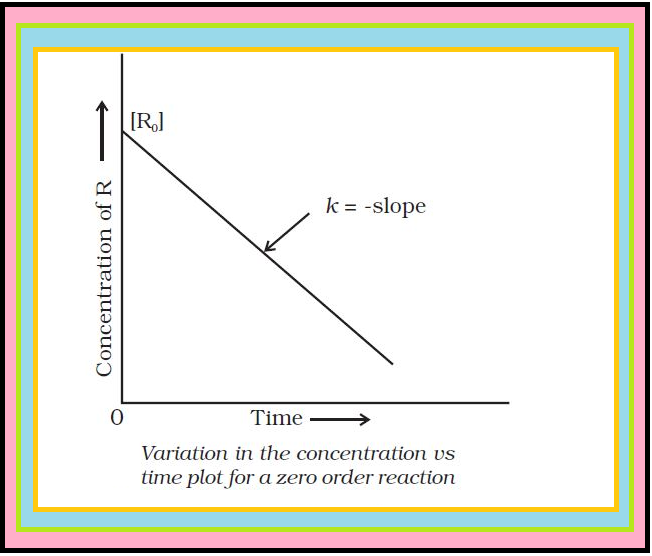
Comparing (2) with equation of a straight line, `color{red}(y = mx + c)`,
if we plot `color{red}([R])` against `color{red}(t)`, we get a straight line (Fig.) with slope `color{red}(= –k)` and intercept equal to `color{red}([R]_0)`.
Further simplifying equation (2), we get the rate constant, `color{red}(k)` as
`color{red}(k = ( [R]_0 - [R])/t)` .................(3)
`=>` Zero order reactions are relatively uncommon but they occur under special conditions.
`=>` Some enzyme catalysed reactions and reactions which occur on metal surfaces are a few examples of zero order reactions.
`=>` The decomposition of gaseous ammonia on a hot platinum surface is a zero order reaction at high pressure
`color{red}(2NH_3 (g) underset (Pt catalyst ) overset(1130 K) → N_2 (g) +3H_2 (g))`.
`color{red}(text(Rate) = k [NH_3]^0 = k)`
● In this reaction, platinum metal acts as a catalyst.
● At high pressure, the metal surface gets saturated with gas molecules. So, a further change in reaction conditions is unable to alter the amount of ammonia on the surface of the catalyst making rate of the reaction independent of its concentration.
`=>` The thermal decomposition of `color{red}(HI)` on gold surface is another example of zero order reaction.
Consider the reaction :
`color{red}(R → P)`
`color{red}(text(Rate) = (- d [ R])/(dt) = k [R]^0)`
As any quantity raised to power zero is unity
`color{red}(text(Rate) = - ( d [R])/(dt) = k xx 1)`
`color{red}(d [R] = - k dt)`
Integrating both sides
`color{red}([R] = - k t +I)` ...............(1)
where, `color{red}(I)` is the constant of integration.
At `color{red}(t = 0),` the concentration of the reactant `color{red}(R = [R]_0)`, where `color{red}([R]_0)` is initial concentration of the reactant. Substituting in equation (1)
`color{red}([R]_0 = - k xx 0 + I)`
`color{red}([R]_0 = I)`
Substituting the value of `color{red}(I)` in in the equation (1)
`color{red}([R] = - k t + [R]_0)` ............(2)
Comparing (2) with equation of a straight line, `color{red}(y = mx + c)`,
if we plot `color{red}([R])` against `color{red}(t)`, we get a straight line (Fig.) with slope `color{red}(= –k)` and intercept equal to `color{red}([R]_0)`.
Further simplifying equation (2), we get the rate constant, `color{red}(k)` as
`color{red}(k = ( [R]_0 - [R])/t)` .................(3)
`=>` Zero order reactions are relatively uncommon but they occur under special conditions.
`=>` Some enzyme catalysed reactions and reactions which occur on metal surfaces are a few examples of zero order reactions.
`=>` The decomposition of gaseous ammonia on a hot platinum surface is a zero order reaction at high pressure
`color{red}(2NH_3 (g) underset (Pt catalyst ) overset(1130 K) → N_2 (g) +3H_2 (g))`.
`color{red}(text(Rate) = k [NH_3]^0 = k)`
● In this reaction, platinum metal acts as a catalyst.
● At high pressure, the metal surface gets saturated with gas molecules. So, a further change in reaction conditions is unable to alter the amount of ammonia on the surface of the catalyst making rate of the reaction independent of its concentration.
`=>` The thermal decomposition of `color{red}(HI)` on gold surface is another example of zero order reaction.